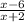Step 1
Given:
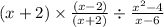
Required: To simplify the problem.
Step 2
Apply PEMDAS (Parentheses, Exponents, Multiplication, Division, Addition, Subtraction).
As a rule, we will move from left to right. This means we will multiply first before dividing.
Therefore for the first part
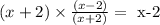
Hence we can write,
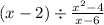
Step 3
Expand x²-4 using the difference of two squares.
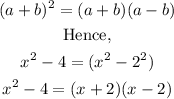
Hence, we can write
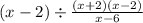
Step 4
Simplify step 3
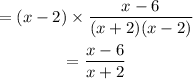
Hence after simplification, the answer is