ANSWER
The volume of iron (III) oxide is 16962.04 Liters
Explanation:
Given information
The density of iron (III) oxide = 5.242 g/cm^3
The mass of iron (III) oxide = 88,915 kg
Let x represents the volume in liters of iron (III) oxide
The first step is to convert the mass from kilograms to grams for unit consistency since the density is already measured in g/cm^3
Recall that, 1 kg is equivalent to 1000 g according to the standard international unit
let x represents the mass to be converted to grams

From the calculation above, you will see that the mass of iron (III) oxide in grams is 88915000 grams.
The next step is to find the volume of iron (III) oxide using the below formula
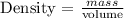
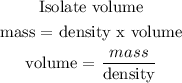
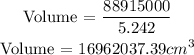
The next step is to convert the cubic centimeters to liters
According to the standard international unit, 1 cm^3 is equivalent to 0.001 L

Therefore, the volume of iron (III) oxide is 16962.04 Liters