To find the slope between those vertices, we can write the lines that contains each pair in slope-intercept form. The line equation in slope-intercept form is:

Where m represents the slope and b the y-intercept.
To find those values we just need to substitute our pair of points on this form and create a system for m and b.
For our first pair of points (0, 1) and (-2, 5), we have
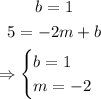
The slope for the first pair is -2.
For the second pair (6, 2) and (4, 6) we have
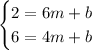
Subtracting the first equation from the first, we get
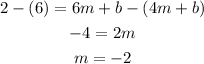
The slope for the other side is also -2.