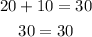The equation for the total points is:

where x is the number of question that worth 3 points and y is the total pionts that worths 4 points. Now we know that in total we can have 30 questions so:
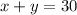
so now we have two incognitas and two equations so we can solve it. so in the secon ecuation we can solve for x so:

now we can substitude x in the first equation like this:
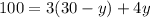
and we can solve for y

Now with the value of y we can replace that in the second equation to find x so
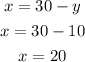
So there will be 20 3-points questions and 10 4-points questions
and we can prove it, replacing the values in the two equation so:
in the first equation:
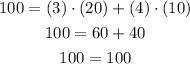
and in the secon equation: