Note that the sum of the angles in a triangle is always 180 degrees.
So it follows that :
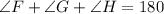
The triangle has a right angle at G, and a right angle measures 90 degrees.
angle F = 4x + 15
and
angle H = 3x + 12
Substitute the angle values to the formula and solve the value of x :
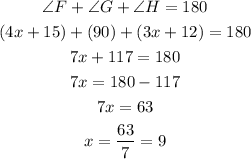
Now we have the value of x, substitute this value to the angles.

Therefore, the angles are :
F = 51 degrees
G = 90 degrees
H = 39 degrees