Answer:
Hypotenuse = 32.0
Step-by-step explanation:
We were given a right angle with the following:
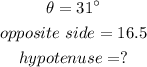
We have one know side, one known angle & one unknown side. To obtain the value of the unknown side, we will use the Trigonometric Ratio (SOHCAHTOA). In this case, we will use "SOH" as shown below:

Therefore, the hypotenuse is 32.0