Question

I need help finding the value of x and y. Then finding the perimeter.

Answer 1

The first thing to note is that the diagonals of any rhombus always bisect each other at a right angle

4x + 3 = 15

4x = 15-3

4x=12

x = 12/4

x = 3

To find y:

In the diagram above,

`\begin{gathered} 17^{2\text{ }}=15^2+h^2 \\ 289=225+h^2 \\ h^2=\text{ 289-225} \\ h^2=64 \\ h=\sqrt[]{64} \\ h=8 \end{gathered}`
`\begin{gathered} 2y\text{ - 4 = h} \\ 2y\text{ - 4 = 8} \\ 2y\text{ = 8+4} \\ 2y=12 \\ y=(12)/(2) \\ y=6 \end{gathered}`

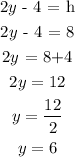
To find the perimeter:

Perimeter of a rhombus = 4L ( where L is length of the one side)

Perimeter = 4 x 17 = 68