Question

Determine the number of solutions for the following system of linear equations. If there is only onesolution, find the solution.5x + 6y + 3z = -13x + 5y + 4z = -5-x - 2y – 2z=5AnswerKeypadKeyboard ShortcutsSelecting an option will enable input for any required text boxes. If the selected option does not have anyassociated text boxes, then no further input is required.O No SolutionO Only One SolutionX =y =z =O Infinitely Many Solutions

Answer 1

We need to solve the next system of equations:

`\begin{gathered} 5x+6y+3z=-1 \\ 3x+5y+4z=-5 \\ -x-2y-2z=5 \end{gathered}`

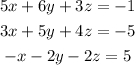
Lets pair equations to eliminate one variable:

`\begin{gathered} 5x+6y+3z=-1 \\ 3x+5y+4z=-5 \end{gathered}`

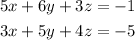
Multiply the first equation by -3 and the second equation by 5:

`\begin{gathered} -3(5x+6y+3z=-1) \\ 5(3x+5y+4z=-5) \end{gathered}`

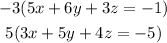
Then, add equations:

`\begin{gathered} -15x-18y-9z=3 \\ 15x+25y+20z=-25 \\ ------------ \\ 0+(-18y+25y)+(-9z+20z)=(3-25) \\ ----------------------- \\ 7y+11z=22 \end{gathered}`

The second pair :

`\begin{gathered} 3x+5y+4z=-5 \\ -x-2y-2z=5 \end{gathered}`

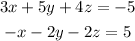
Multiply the first equation by 1 and the second equation by 3:

`\begin{gathered} 1(3x+5y+4z=-5) \\ 3(-x-2y-2z=5) \\ \\ \end{gathered}`

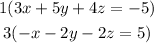
Add both equations:

`\begin{gathered} 3x+5y+4z=-5 \\ -3x-6y-6z=15 \\ ------------- \\ 0+(5y-6y)+(4z-6z)=-5+15 \\ ------------------------ \\ -y-2z=10 \end{gathered}`

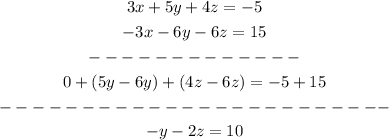
Solve the new system:

`\begin{gathered} 7y+11z=22 \\ -y-2z=10 \end{gathered}`

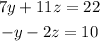
Multiply the first equation by 1 and the second equation by 7:

`\begin{gathered} 1(7y+11z=22) \\ 7(-y-2z=10) \\ \\ \end{gathered}`

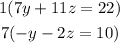
Add both equations:

`\begin{gathered} 7y+11z=22 \\ -7y-14z=70 \\ ------------- \\ 0+(11z-14z)=-22+70 \\ ---------------- \\ -3z=48 \\ z=-16 \end{gathered}`

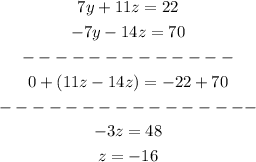
Replace the z value on one equation:

`-y-2(-16)=10`

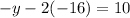
Solve for y:

`\begin{gathered} -y+32=10 \\ \\ -y=10-33 \\ y=22 \end{gathered}`

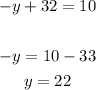
Finally, replace the y value and the z value:

`\begin{gathered} -x-2y-2z=5 \\ -x-2(22)-2(-16)=5 \\ -x-44+32=5 \\ -x-12=5 \\ -x=5+12 \\ x=-17 \end{gathered}`

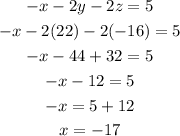
Hence, the result for the variables are:

x=-17

y=22

z=-16