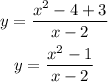Question

4d) write the equation of a rational function with the given characteristics.Vertical asymptote at x = 2, slant asymptote at y =× + 2, passes through the point (3,8)

Answer 1

The rational function will have the next form:

`y=(p(x))/(d(x))`

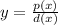
where p(x) and d(x) are two polynomials.

Given that the function has a vertical asymptote at x = 2, then d(x) must have a zero at x = 2. Since this is the only asymptote, then:

`d(x)=x-2`

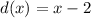
A slant asymptote is present when the degree of the polynomial of the numerator is exactly one more than the degree of the denominator. This means that p(x) must be a polynomial a degree 2.

The quotient, q(x), between p(x) and d(x) is the equation of the slant asymptote. The next relation must be satisfied:

`p(x)=d(x)\cdot q(x)+r(x)`

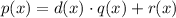
where r(x) is the remainder of the division. Assuming the remainder is a constant, k, and substituting with d(x) = x-2 and q(x) = x + 2, the slant asymptote, we get:

`\begin{gathered} p(x)=(x-2)(x+2)+k \\ p(x)=(x^2-2^2)+k \\ p(x)=x^2-4+k \end{gathered}`

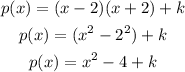
We know that the curve passes through the point (3, 8), that is, when x = 3, then y = 8. Substituting with this point and the functions p(x) and d(x), we get:

`\begin{gathered} y=(x^2-4+k)/(x-2) \\ 8=(3^2-4+k)/(3-2) \\ 8=(5+k)/(1) \\ 8-5=k \\ 3=k \end{gathered}`

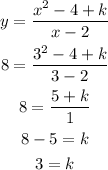
Finally, the rational function is:

`\begin{gathered} y=(x^2-4+3)/(x-2) \\ y=(x^2-1)/(x-2) \end{gathered}`