Question

Which of the following equationns describes this graph?

Answer 1

Step-by-step explanation: The provided is the graph of a parabola, therefore it can be modeled by the vertex form of the standard equation of the parabola, which is as follows:

`\begin{gathered} y(x)=A(x-B)^2+C\Rightarrow(1) \\ \\ (B,C)=(x,y)\Rightarrow\text{ Vertex} \end{gathered}`

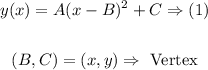
According to the graph, the vertex of the parabola is:

`(x,y)=(2,-2)`

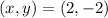
Plugging it in equation (1) results in the following:

`y(x)=A(x-2)^2-2`

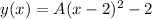
The value of the coefficient A can be determined by plugging in the coordinates of the point (4,2) in the above equation:

`\begin{gathered} 2=A(4-2)^2-2 \\ \\ 4=A(2)^2 \\ \\ 4=4A \\ \\ A=1 \end{gathered}`

Therefore the equation that describes the graph is as follows:

`y(x)=(x-2)^2-2\Rightarrow\text{ Option\lparen A\rparen}`

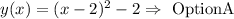
The graph confirmation: