From the given triangle, the dimensions are
![\begin{gathered} XY=110\operatorname{mm}=z \\ YZ=141\operatorname{mm}=x \\ XZ=203\operatorname{mm}=y \end{gathered}]()
To find the unknown angle, we find angle m∠Y
Using the cosine rule formula which is shown below
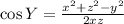
Where
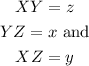
Substitute the values of x, y and z into the cosine rule formula
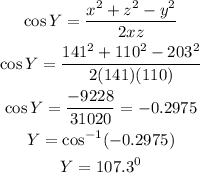
Let the unknown angle be k, since m∠Y is known,
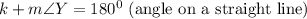
Substitute 107.3° of m∠Y into the formula above
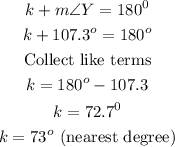
Hence, the unknown angle 73^