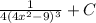Question

a) What is the inside function? What is its derivative?b) Perform the integration using u-substitution.

Answer 1

Given the integral function below

`\int(-6x)/((4x^2-9)^4)dx`

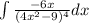
a) The inside function is

`\begin{gathered} 4x^2-9 \\ \end{gathered}`
`\therefore u=4x^2-9`

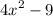
The derivative of the inside function is

`\begin{gathered} u^(\prime)=8x \\ (du)/(dx)=8x \\ dx=(du)/(8x) \end{gathered}`

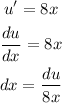
b) Therefore, the integral can be written as:

`\int(-6x)/((4x^2-9)^4)dx\rightarrow\int(-6x)/(u^4)*(du)/(8x)`
`\rightarrow\int(-3)/(4u^4)du\rightarrow-(3)/(4)\int u^(-4)du`
`\begin{gathered} \rightarrow-(3)/(4)((u^(-4+1))/(-4+1))+C \\ \rightarrow-(3)/(4)*(u^(-3))/(-3)+C \\ \rightarrow(1)/(4)*(1)/(u^3)+C \end{gathered}`
`\begin{gathered} (1)/(4u^3)+C \\ Recall\text{ u=}4x^2-9 \\ \therefore \\ \int(-6x)/((4x^2-9)^4)dx=(1)/(4(4x^2-9)^3)+C \end{gathered}`

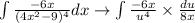
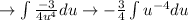
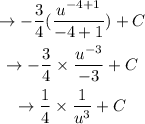
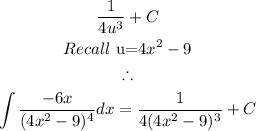
Final answer:

a) The inside function is 4x² - 9, and the derivative is 8x

b) The integration by substitution is

`\begin{equation*} (1)/(4(4x^2-9)^3)+C \end{equation*}`