the Given the geometry of the wall as shown below
The wall takes the shape of a trapezoid.
The unpainted door labeled as A takes the shape of a rectangle.
The unpainted window labeled as B takes the shape of a triangle.
The area of the wall painted by Marcus is evaluated as
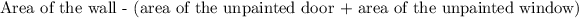
Area of the wall:
Recall that the wall takes the shape of a trapezoid. Thus, the area of a trapezoid is evaluated as

Thus, the area of the wall becomes
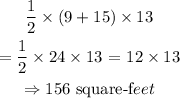
Area of the unpainted door:
Recall that the unpainted door, labeled as A, takes the shape of a rectangle. Hence, the area of a rectangle is evaluated as

The area of the unpainted door becomes
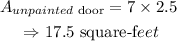
Area of the unpainted window:
Recall that the unpainted window, labeled as B, takes the shape of a triangle. Thus, the area of a triangle is evaluated as

Thus, the area of the unpainted window becomes
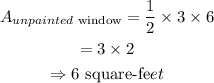
Thus, the area of the painted wall becomes

Hence, the area of the wall painted by Marcus is 132.5 square-feet