Step 1
State the formula for exponential growth
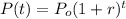
where;
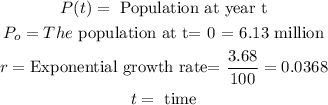
Step 2
A) Find the exponential growth function
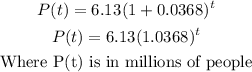
Step 3
B) Estimate the population of the city in 2018
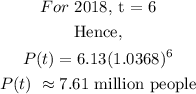
Step 4
C) When will the population of the city be 10million

Step 5
Find the doubling time
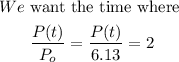
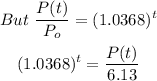

The doubling time approximately to 1 decimal place = 19.2 years