We will find the linear equation of the graph shown. For doing so, we will find the slope with the formula:

Where (x₁, y₁) and (x₂, y₂) are the coordinates of two points of the line.
In this line, we see that the graph passes through the points:
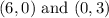
This gives us the y-intercept, as the function passes for y=3, when x=0. The slope is given by:
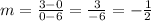
Hence, the linear equation of the function shown is:
