Question

What is the equation of the quadratic function that has a minimum at 7 or -3 and goes through 9 9

Answer 1

Given the information about the coordinates which represents the minimum point for the function, and a point that it passes through, obtaining the equation that represents the function is easy.

For a quadratic function that is represented by the general form:

`f(x)=ax^2+bx+c`

The formula that gives the x-coordinate for the minimum point of a quadratic function is given as:

`x=-(b)/(2a)`

Now, since the minimum point is (7, -3) with the x-coordinate being x =7, we have:

`\begin{gathered} x=-(b)/(2a) \\ 7=-(b)/(2a) \\ 7*2a=-b \\ 14a=-b \\ b=-14a\ldots\ldots...\ldots\ldots\ldots\text{.}\mathrm{}(1) \end{gathered}`

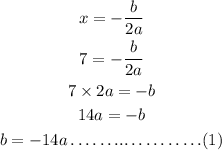
Since the curve passes through (9,9) we have that f(x)=9, x = 9, thus:

`\begin{gathered} \text{From: f(x) = ax}^2+bx+c \\ 9=a(9)^2+b(9)+c \\ 9=\text{ 81a+9b+c }\ldots\ldots\ldots\ldots\ldots\ldots..(2) \end{gathered}`

Now, using the minimum point again, (7, -3), where f(x)= -3. and x = 7, we have :

`\begin{gathered} f(x)=ax^2+bx+c \\ -3=a(7)^2+b(7)+c \\ -3=49a+7b+c\ldots\ldots\ldots\ldots\ldots\ldots\ldots.(3) \end{gathered}`

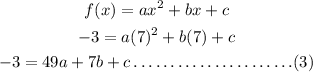
Now, we will solve these three equations simultaneously, as follows:

Substitute the value of b in equation (1) into equations (2) and (3), as follows:

`\begin{gathered} \sin ce\colon\text{ b=-14a} \\ \text{from equation 2: }9=\text{ 81a+9b+c }\ldots\ldots\ldots\ldots\ldots\ldots..(2) \\ 9=\text{ 81a +9(-14a) + c} \\ 9\text{ = 81a-126a + c} \\ 9=-45a+c\ldots\ldots\text{...}.(2) \\ \text{From equation 3: }-3=49a+7b+c\ldots\ldots\ldots\ldots\ldots\ldots\ldots.(3) \\ -3=49a+7(-14a)+c \\ -3=49a-98a+c \\ -3=-49a+c\ldots\ldots\ldots\text{.(3)} \end{gathered}`

Now, solving equations (2) and (3) gives:

`\begin{gathered} 9=-45a+c\ldots\ldots\text{...}.(2) \\ -3=-49a+c\ldots\ldots\ldots\text{.(3)} \\ \text{subtracting (3) from (2)} \\ 9-(-3)=-45a-(-49a)+\text{ c-c} \\ 9+3\text{ =-45a+49a} \\ 12=4a \\ a=(12)/(4)=3 \\ \text{substitute for a in }(2) \\ 9=-45a+c \\ 9=-45(3)+c \\ 9=-135+c \\ c=\text{ 9+135=144} \end{gathered}`

Now, we use equation (1) to obtain the value of b, as:

`\begin{gathered} \text{from (1): b=-14a} \\ \sin ce\text{ a = 3} \\ b=\text{ -14(3)= -42} \end{gathered}`

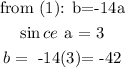
Therefore the equation of the function f(x) is:

`\begin{gathered} f(x)=ax^2+bx+c \\ f(x)=(3)x^2+(-42)x+144 \\ f(x)=3x^2-42x+144 \\ In\text{ vertex form, this is expr}essed\text{ as:} \\ f(x)=3(x-7)^2-3 \end{gathered}`

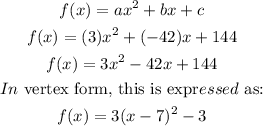
Thus, 3 goes into the first blank at the far left, 7 goes into the middle blank. and -3 goes into the last blank at the far right