Question

I don't understand this questions please explain in simple words

Answer 1

`(2)/(5)x\text{-3=-17}`

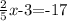
To solve the equation, you must put the variable on one side and the other terms on the other side, then make its coefficient equal to 1

Add 3 to both sides

`\begin{gathered} (2)/(5)x-3+3=-17+3 \\ (2)/(5)x=-14 \end{gathered}`

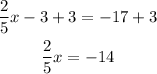
Now multiply both sides by 5 to cancel the denominator on the left side

`\begin{gathered} (2)/(5)x*5=-14*5 \\ 2x=-70 \end{gathered}`

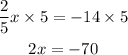
Divide both sides by 2 to find x

`\begin{gathered} (2x)/(2)=-(70)/(2) \\ x=-35 \end{gathered}`

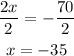
The solution of the equation is x = -35

The answer of no. 44 is x = -35