Answer:
The parent function is given below as
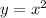
Step 1:
For the function to be shifted 2 units to the left, we will have the equation below
The function h(x) = f(x + a) represents a horizontal shift a units to the left. Informally: Adding a positive number after the x inside the parentheses shifts the graph left,
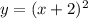
The graph is represented below as
Step 2:
The function above is then shifted 10 units down, this can be represented with the function below
When a function shifts vertically, the y-value changes. The x-value stays the same, while the y-value changes the amount of the shift. If it shifts up, then we add the value to the y-term. If it shifts down, we will subtract that value from the y-term.
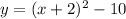
The function is represented below as
Step 3:
The function above is then flipped to open downwards
Reflections. Parabolas can also be reflected in the x-axis. Thus the parabola y=−x2 is a reflection of the basic parabola in the x-axis.
Hence,
The function will be
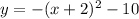
Hence,
The graph is given below as
Therefore,
The function that defines the curve will be given below as
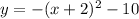
The graph is given below as
Hence,
The final answer is y = -(x+2)²- 10