Question

How much time must you invest $8500 at 6.25% compounded continuously tohave $13840.04? Round the time t to 2 decimal places.

Answer 1

Write out the compound interest formula

`A=Pe^(rt)`

Where A= The final Amount after the compound interest

P= The initial amount invested

r=rate

t=time

Write out the given parameters in the question

`\begin{gathered} P=\text{ \$8500} \\ r=6.5\text{ \%} \\ t=\text{unknown} \\ A=\text{ \$13840.04} \\ e=2.71828 \end{gathered}`
`\begin{gathered} 13840.04=8500* e^(0.0625t)^{} \\ e^(0.0625t)=(13840.04)/(8500) \\ e^(0.0625t)=1.62824 \end{gathered}`
`\begin{gathered} 0.0625t=In(1.62824) \\ 0.0625t=0.4875997 \end{gathered}`
`\begin{gathered} t=(0.4875997)/(0.0625) \\ t=7.79999483 \end{gathered}`
`t=7.80(\text{nearest 2 decimal places)}`

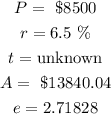
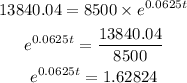
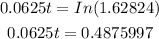
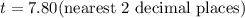
Hence, the time required for the compounded continuous investment is 7.80