The circumcenter can be found as the intersection of the perpendicular bisectors.
The middle points of each one of the segments are
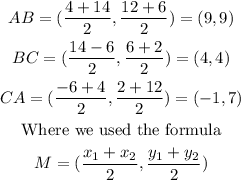
Furthermore, the slopes of each one of the three segments are
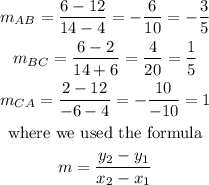
In general, two lines are perpendicular if the product of their slopes is equal to -1. Then, the slopes of a perpendicular line to each one of the segments are

Given a point on a line and its slope, we can calculate the equation of any line. In our case,
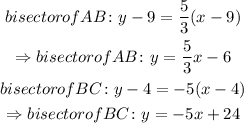
Finally, calculate the intersection point of lines AB and BC as shown below
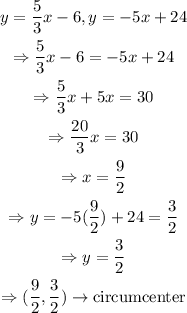
The answer is (9/2,3/2)
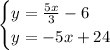
Remember that (properties of equality)
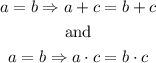
From the system of equations,
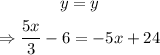
Then, add +6 to both sides of the equation,
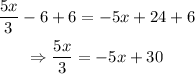
Add +5x to both sides of the equation,
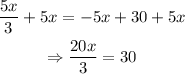
Multiply both sides of the equation by 3
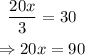
Finally, multiply both sides of the equation by 1/20
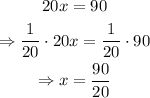
There was no need to use negative reciprocals.