Answer:
$2186.17
Step-by-step explanation:
The amount in the account after 2 years can be calculated using the following equation:

Where P is the initial amount, r is the interest rate, n is the number of times the interest is compound per year and t is the number of years.
The interest rate is 4 1/2 % which is equivalent to 4.5% or 0.045 because:
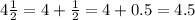
Then, replacing P by $2,000, r by 0.045, n by 2, and t by 2 years, we get:

Therefore, after 2 years there will be $2186.17 in the account.