ANSWER
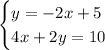
Step-by-step explanation
A system of two equations with infinite solutions is a system which equations both represent the same line.
For example, if we have the line y = -2x + 5, a product of this equation and any number will make another equation representing the same line. We can multiply it by 2 and have: 2y = -4x+10.
Hence, a system of equations could be:
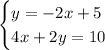
The first equation is in slope-intercept form and the second equation is in standard form