Question

You are standing 400 feet from the base of a launch pad. If a rocket is launched vertically at a constant speed of 2000 feet per second, at what angle of elevation will you see the rocket after 3 seconds? What about after 10 seconds?Show your work.

Answer 1

1) Gathering the data

400 feet ----- base of a launch pad

rocket is launched vertically

Speed: 2000 ft per second

A) Angle of elevation of the rocket after 3 seconds?

Let's visualize that

Considering that V= 200 ft/second

200ft ---------- 1 second

x --------------- 3

x= 600 ft

Angle of elevation tan (θ), so with that info let's find the angle with arctang(θ)

`\begin{gathered} \tan (\theta)\text{ =}(600)/(400) \\ \tan (\theta)=(3)/(2) \\ \theta=\tan ^(-1)((3)/(2)) \\ \theta=56.309 \end{gathered}`

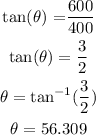
B)

200 ----------- 1

y ----------------10

y=2000 ft

`\begin{gathered} \tan (\theta)\text{ =}(2000)/(400) \\ \tan (\theta)\text{ =}5 \\ \theta=\tan ^(-1)(5)\text{ =78.69} \end{gathered}`

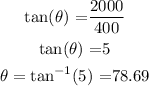
3) So the angles are: A) 56.30º after 3 seconds and B) 78.69º after 10 seconds