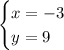Question

X+4y=332y+6x=0Solve the equations algebraically

Answer 1

We want to solve the following system

`\begin{cases}x+4y=33 \\ 2y+6x=0\end{cases}`

We can start by dividing the second equation by 2

`\begin{cases}x+4y=33 \\ y+3x=0\end{cases}`

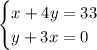
Then, if we subtract 3x from both sides of the second equation, we're going to have

`\begin{cases}x+4y=33 \\ y=-3x\end{cases}`

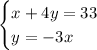
If we substitute the expression for y on the second equation by the y on the first equation, we get a new equation only for x.

`\begin{gathered} x+4(y)=33 \\ x+4(-3x)=33 \\ x-12x=33 \\ -11x=33 \\ x=-(33)/(11) \\ x=-3 \end{gathered}`

Using this values for x on the second equation, we find the value for y.

`\begin{gathered} y=-3(x) \\ y=-3(-3) \\ y=9 \end{gathered}`

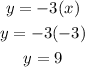
And those are the solutions for this system.

`\begin{cases}x=-3 \\ y=9\end{cases}`