Question

A golf ball m=0.155 kg strikes a vertical concrete wall elastically with a horizontal velocity of v = 13 m/s.Randomized Variablesm = 0.155 kg a) write an expression for the magnitude of the impulse experienced by the ball b)if the ball was in contact with the wall for .1s what is the magnitude of the force the ball experienced in N? C) if the ball rebounds with only half the velocity it comes with, what is the magnitude of the impulse in kg m/s? D) determine the magnitude of the change in the kinetic energy of the ball when it rebounds at half speed. Give your answers in joules. v = 13 m/s

Answer 1

a)

The impulse is given as the change in momemtum, that is:

`\begin{gathered} I=\Delta p \\ \text{ where} \\ p=mv \\ \text{ then} \\ I=mv_f-mv_i \\ I=m(v_f-v_i) \end{gathered}`

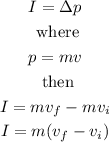
Therefore, the expression for the impulse is:

`I=m(v_f-v_i)`

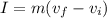
b)

We know that the average force is related to the impulse by:

`F=(I)/(t)`

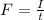
We know that the ball has an initial velocity of 13 m/s and since the colission is elastic the velocity has to be the same but pointing to the other direction, then the final velocity is -13 m/s (assuming the positive direction is the initial direction of motion). Then we have:

`\begin{gathered} F=((0.155)(-13-13))/(0.1) \\ F=-40.3 \end{gathered}`

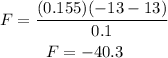
Since we are looking for the magnitude we drop the sign; therefore, the force is 40.3 N.

c)

In this case the final velocity is -6.5 m/s, then the impulse is:

`\begin{gathered} I=(0.155)(-6.5-13) \\ I=-3.0225 \end{gathered}`

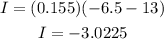
Therefore, the magnitude of the impulse in this case is 3.0225 kg m/s.

d)

The change in kinetic energy is:

`\begin{gathered} (1)/(2)mv_f^2-(1)/(2)mv_i^2=(1)/(2)(0.155)(-6.5)^2-(1)/(2)(0.155)(13)^2 \\ =-9.823125 \end{gathered}`

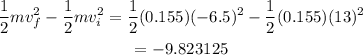
Therefore, the magnitude of the change in kinetic energy is 9.823125 J