Question

Find the compound interest $82,000 at 10% compounded semiannually for 4 years

Answer 1

• Total amount: $121,151.35

,

• Interest earned: $39,151.35

Step-by-step explanation

The amount that will be in the account is,

`A=P\mleft(1+(r)/(n)\mright)^(nt)`

Where:

• A: total amount

,

• P: principal amount = $82,000

,

• r: annual interest rate = 0.10

,

• n: number of times compounded per year = 2

,

• t: time in years = 4

Input this information in the equation above,

`A=82,000\mleft(1+(0.10)/(2)\mright)^(2\cdot4)=82,000\mleft(1.05\mright)^8\approx121,151.35`

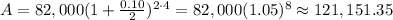
The total amount that will be in the account after 4 years is $121,151.35, rounded to the nearest cent.

The interest earned is the difference between the total amount after the given period of time and the principal amount,

`i=A-P=121,151.35-82,000=39,151.35`

Hence, the total interest earned in those 4 years was $39,151.35