In order to find the point C, which is located at 2/5 of the distance, we can calculate separately the x and y coordinates.
For the x-coordinate, the horizontal distance is the x-coordinate of the second point minus the x-coordinate of the first point:
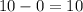
Now we just need to find 2/5 of this distance:
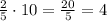
So the x-coordinate of the point C is 4.
Now, calculating the y-coordinate, the vertical distance is the y-coordinate of the second point minus the y-coordinate of the first point:
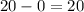
Now, calculating 2/5 of this distance, we have:
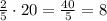
So the y-coordinate of point C is 8.
Therefore the coordinates of point C are (4, 8)