Given:
Tory watches a cheetah as it sits on the top of a cliff.
The line of sight to the cheetah forms a 33° angle of elevation.
The telescope sits 2.4 m above the ground.
The base of the telescope is 193 m from the base of the cliff.
Required:
We need to ind the height of hthe cliff.
Step-by-step explanation:
The Height of the cliff is BC.
We need to find the measure of BC.
We know that AD=AB=193m, and T=BD=2.3m.
Let DC=x m.
Consider the tan fomula.
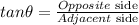
Substitute opposite side= DC=x and adjacent side = AD=193m in the formula.
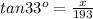

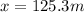
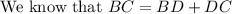
Substitute BD =2.4 m and DC=125.3 m in the equation.
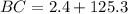
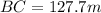
Fial answer:
The cheetah sits on the top of a cliff from 127.7m of the ground.