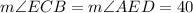Question

In the rectangle below, AE = 4x + 6, BD=5x+30, and m L ECB=40°Find the value of x and m 4 AED.

Answer 1

Hello!

Let's write some important information:

AE = 4x +6

BD = 5x +30

ECB = 40º

AED = ?

First, note that AE is half AC. So, we know that:

`\begin{gathered} AC=2(AE) \\ AC=2(4x+6) \\ \boxed{AC=8x+12} \end{gathered}`

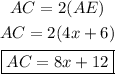
Now, other important information:

AC is equal to BD, so we can obtain the value of x:

`\begin{gathered} AC=BD \\ 8x+12=5x+30 \\ 8x-5x=30-12 \\ 3x=18 \\ x=(18)/(3) \\ \boxed{x=6} \end{gathered}`

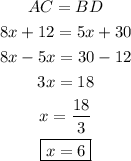
Now, let's remember the definition of vertex opposite angles:

• the sides of these angles are opposite lines and do not share any of the sides;

• these angles are congruent (equal).

So, we also can say that:

`m\angle ECB=m\angle AED=40\degree`