Question

A fair coin is flipped 7 times. What is the probability of flipping 4 tails? Round the answer to thenearest hundredth of a percent.

Answer 1

The coin is flipped 7 times i.e n=7

The probability of getting tails =1/2 on single toss

and probability of getting head=1/2

The total number of possible outcomes is 2^7

`\begin{gathered} =2^7 \\ =128 \end{gathered}`

Total number of possible outcomes=128.

Probability getting 4 tails in total 7 tossed,

Apply combination expression:

`^nC_r=(n!)/(r!(n-r)!)`

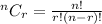
Here we have, n=7, r=4

`\begin{gathered} ^7C_4=(7!)/(4!(7-4)!) \\ ^7C_4=(7!)/(4!*3!) \\ ^7C_4=(7*6*5*4!)/(4!*3*2*1) \\ ^7C_4=(35)/(1) \\ ^7C_4=35 \end{gathered}`

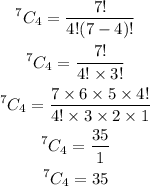
So, the probability of flipping 4 tails out of 7 toss is = 35/128

Percent probabilty=

`\begin{gathered} P=(35)/(128)*100 \\ P=27.34\text{ percent} \end{gathered}`

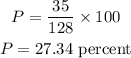
The probability is 27.34%