Question

What are the degrees of freedom for a Pearson's correlation coefficient if thecovariance of x and y = 4, and the sample size = 250?

Answer 1

Pearson's correlation coefficient (r):

The Pearson's correlation coefficient is a measure of how strong/weak is the association between two variables.

It also tells us the direction of the relationship (positive/negative)

The degrees of freedom for a Pearson's correlation coefficient can be found as

`df=n-2`

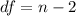
Where n is the sample size.

For the given case, the sample size is 250.

So, the degree of freedom is

`\begin{gathered} df=n-2 \\ df=250-2 \\ df=248 \end{gathered}`

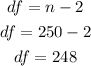
Therefore, the degrees of freedom for the Pearson's correlation coefficient is 248