Question

I need help with a problem Find the equation of the line containing given points (3,2) and (-4, -8)

Answer 1

y = (10/7)x - 16/7

Step-by-step explanation:

The equation of a line that passes through two points (x1, y1) and (x2, y2) is

`\begin{gathered} y-y_1=m(x-x_1) \\ \\ \text{ Where} \\ m=(y_2-y_1)/(x_2-x_1) \end{gathered}`

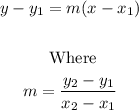
So, replacing (x1, y1) = (3, 2) and (x2, y2) = (-4, -8), we get:

`m=(-8-2)/(-4-3)=(-10)/(-7)=(10)/(7)`
`y-2=(10)/(7)(x-3)`

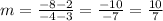
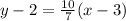
Finally, we can solve the equation for y

`\begin{gathered} y-2=(10)/(7)x-(10)/(7)(3) \\ \\ y-2=(10)/(7)x-(30)/(7) \\ \\ y=(10)/(7)x-(30)/(7)+2 \\ \\ y=(10)/(7)x-(16)/(7) \end{gathered}`

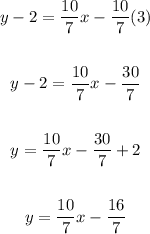
Therefore, the answer is

y = (10/7)x - 16/7