Question

Let P(x)=ln(4x-5)^k for some positive-valued constant k. If P'(2)=8, what is the value of k?

Answer 1

The expression we have is:

`P(x)=ln(4x-5)^k`

First, we use the following property of natural logarithms:

`\ln A^k=k\ln A`

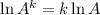
the exponent is lowered.

We apply this to our expression:

`P(x)=k\ln (4x-5)`

Next, we use the given condition:

`P(2)=8`

What this means is that when x=2, the value of P(x) is 8.

We need to substitute this two values into our expression:

`\begin{gathered} P(x)=k\ln (4x-5) \\ 8=k\ln (4(2)-5) \end{gathered}`

We solve the expression inside the natural logarithm:

`\begin{gathered} 8=k\ln (8-5) \\ 8=k\ln (3) \end{gathered}`

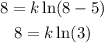
Now, since ln(3)=1.1 we get:

`8=k(1.1)`

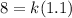
We solve for k by dividing both sides by 1.1:

`\begin{gathered} (8)/(1.1)=k \\ 7.3=k \end{gathered}`

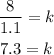
The value of k is 7.3