Question

A population doubles in 15 years. By what percentage is the population growing each year?

Answer 1

The population growth formula is :

`P=P_oe^(rt)`

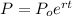
where P = total population after time t

Po = initial population

e = euler's number

r = percent rate of growth

t = time in years

From the problem, the population doubles in 15 years, so we have :

P = 2Po

t = 15

Using the formula above :

`\begin{gathered} 2P_o=P_oe^(15r)_{} \\ 2=e^(15r) \end{gathered}`

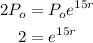
Take ln of both sides :

`\ln 2=\ln (e^(15r))`

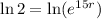
Note that :

`\begin{gathered} \ln a^m=m\ln a \\ \text{and} \\ \ln e=1 \end{gathered}`

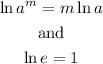
The natural logarithm of a raised to m is the same as m multiplied by the natural logarithm of a.

So the equation will be :

`\begin{gathered} \ln 2=15r\ln e \\ \ln 2=15r \\ r=(\ln 2)/(15) \\ r=0.0462 \end{gathered}`

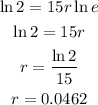
The answer is 0.0462 or 4.62%