1 Answer

Matm · Answer 1 · 2023-11-17T03:45:28+0000

The volume of a hemisphere is given by:

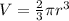
$V=(2)/(3)\pi r^3$

where r is its radius.

In this case we know that the diameter is 48 in and we know that the radius is half the diameter, then we have:

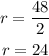
$\begin{gathered} r=(48)/(2) \\ r=24 \end{gathered}$

Plugging the value in the formula for the volume we have:

$\begin{gathered} V=(2)/(3)\pi(24)^3 \\ V=(2)/(3)\pi(13824) \\ V=9216\pi \end{gathered}$

Therefore, the volume of the hemisphere is:

$V=9216\pi\text{ cubic inches}$

Please log in or register to add a comment.