Question

Consider the function f(x) = 8x - x^2Compute the derivative values of the following:f'(0):f'(1) =f'(2) =f'(3) =Conjecture a formula for f'(a) that depends only on the value a. That is, in the same way that we have a formula for f(x) (recall f(x) = 8x- x^2), see if you can use your work above to guess a formula for f'(a) in termsof a.f'(a)=

Answer 1

Question:

Solution:

Consider the following function:

`f(x)=8x-x^2`

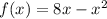
The derivative of this function is:

`f^(\prime)(x)=8-2x^{}`

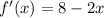
Now, if we evaluate this function at x = 0, x=1, x= 2 and x= 3, we get respectively:

`f^(\prime)(0)=8-2(0)=8-0=8^{}`

`f^(\prime)(1)=8-2(1)=8-2=6^{}`

`f^(\prime)(2)=8-2(2)=8-4=4^{}`

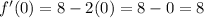
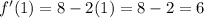
and

`f^(\prime)(3)=8-2(3)=8-6=2^{}`

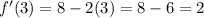
According to this:

`f^(\prime)(a)=8-2(a)^{}`

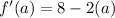
So that, we can conclude that the correct answers are:

`f^(\prime)(0)=8^{}`

`f^(\prime)(1)=6^{}`

`f^(\prime)(2)=4^{}`

`f^(\prime)(3)=2^{}`

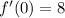
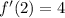
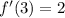
and

`f^(\prime)(a)=8-2a`

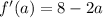
Note that the above formula is a function of a, so it depends only of a.