We have to find the coordinates of point P.
It is located in the segment AB and it is located such as the ratio AP:PB is 3:2.
We can think of the ratio as if the segment has 3+2=5 equally sized subsegments, where 3 subsegments are the distance from A to P and 2 subsegments are the distance from P to B:
As we have A and B located in certain coordinates of xy-plane, we can use the fact that P is goin to be 3/5 of the distance between AB in the x-coordinates and also in the y-coordinates, so we can calculate each coordinate separately:

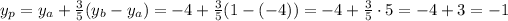
The coordinates of P are