0. Please, check below.
,
1. scalene triangle and not right
1) Let's find the lengths of the sides of this triangle so that we can classify this triangle.
1.2) And for the slope of each side, we can plug the coordinates into the slope formula:
Slopes:
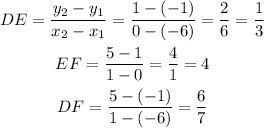
2) We can use the distance formula:
![\begin{gathered} d=\sqrt[]{(y_2-y_1)^2+(x_2-x_1)} \\ d_(EF)=\sqrt[]{(5-1)^2+(1-0)^2}=\sqrt[]{17} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/igmh6cosknms2m4f6pwhrizspp92o4pudq.png)
Note that we have plugged the coordinates into that, let's proceed with that to find the two other legs' length:
![\begin{gathered} d_(DE)=\sqrt[]{(1-(-1))^2+(0-(-6))^2}=\sqrt[]{40}=2\sqrt[]{10} \\ d_(DF)=\sqrt[]{(5-(-1))^2+(1-(-6))^2}=\sqrt[]{85} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/qhtxoeow7wuswbjo8dx3rrkc5hme8nq7wj.png)
![\begin{gathered} \text{length:} \\ DE=\sqrt[]{40} \\ EF=\sqrt[]{17} \\ DF=\sqrt[]{85} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/ahyt95j5fojov0ndwu5miaguxbyn2d3osq.png)
2.2) Note that this is a scalene triangle due to those unequal side lengths. We can check whether this is a right triangle using the Pythagorean Theorem:
![\begin{gathered} a^2=b^2+c^2 \\ (\sqrt[]{85})^2=(\sqrt[]{17})^2+(\sqrt[]{40})^2 \\ 85\\e17+40 \\ 85\\e57 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/2wfo4si48mg0g36q9ibwnn9kkzxx0fk7bp.png)
Plugging into the largest side, we can see that it does not verify so this is a scalene triangle and not right