Question

I need help on a problem

Answer 1

To simplify the radical, factorize the number under it using the prime number

Since 252 is an even number, then let us divide it by the 1st prime number 2

`(252)/(2)=126`

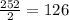
since 126 is an even number, then divide it by 2 again

`(126)/(2)=63`

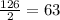
Now 63 is an odd number and sum of its digit = 6 + 3 = 9, then

Divide it by the 2nd prime number 3

`(63)/(3)=21`

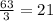
Since 21 is divisible by 3, divide it by 3 again

`(21)/(3)=7`

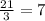
Then 252 = 2 x 2 x 3 x 3 x 7, put them under the radical

`\sqrt[]{252}=\sqrt[]{2*2*3*3*7}`

Each number repeated twice can go out the radical, then

2 and 3 will go out the radical

`\begin{gathered} \sqrt[]{252}=2*3*\sqrt[]{7} \\ \sqrt[]{252}=6\sqrt[]{7} \end{gathered}`

The simplest form of the radical is 6 square root 7

`\begin{gathered} \sqrt[]{252}=\sqrt[]{4}*\sqrt[]{63} \\ =2*\sqrt[]{63} \\ =2*\sqrt[]{9}*\sqrt[]{7} \\ =2*3*\sqrt[]{7} \\ =6*\sqrt[]{7} \\ =6\sqrt[]{7} \end{gathered}`