Part A. We are given that the deformation of a string from its equilibrium position is given by the following equation:
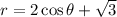
To determine the value of the angle for which the spring in at equilibrium we need to set the equation to zero:
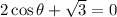
Now, we solve for the time. First, we subtract the square root of 3 to both sides:
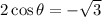
Now, we divide both sides by 2:
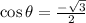
now, we take the inverse function of the cosine:
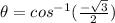
Solving the operation:
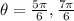
This two angles apply for the interval of time:
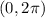
Part B. Now, we are asked to double the angle. We get the following equation:

Now, we set the equation to zero:

Now, we solve for the time similarly as we did in the previous point:

Now, we solve the right side:
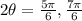
Dividing both sides by 2:
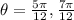
In this case, for the interval between 0 and 2 pi we have that the possibe values of time are:
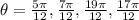
The difference with the values in part A is that the spring now passes through the equolibrium point at a greater frequency.
Part C. We are given that another spring has the following equation :
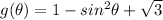
To determine the value of time where the spring are at equilibrium we set both equations equal:

We can cancel out the square root of 3:
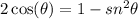
Now, we use the following trigonometric identity:
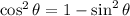
Substituting we get:

Now, we subtract the left side:
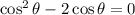
Now, we take cosine as common factor:
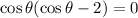
Now, we set each factor to zero:
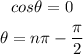
For:
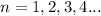
For the second factor we have:
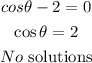
Therefore, the times are the solution to the first factor.