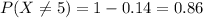Answer: 0.86
Step-by-step explanation
The experimental probability P is found by:
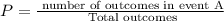
As we want to know the experimental probability of rolling a number other than 5, we can find the probability of rolling 5 and subtract it to 1. Thus, the number of outcomes we got of 5 is 14, while the total outcomes is the sum of all:
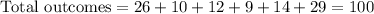
Then, the P(X=5) is:
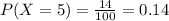
Finally, the P(X≠5) is: