The shape that is shown in the image is a heptagon (it has 7 sides), to determine the sum of the interior angles of any shape you have to subtract 2 to the number of sides of the shape and multiply the result by 180. The formula is as follows:
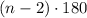
Where n represents the number of sides.
The sides on the heptagon are n=7, replace this value in the formula and you get the sum of the inner angles of the shape:
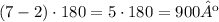
The sum of the interior angles of the heptagon is 900º
Now that you know how much do the interior angles add up to we can calculate the value of x as follows:
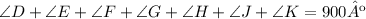
Replace the expression with the known measures of all angles and calculate for the missing one
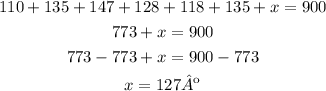
The value of x is 127º