Question

Two gardeners can do the weekly yard maintenance is 30 minutes if they work together. The older gardener takes 28 minutes more than the younger gardener to finish the job my himself. How long does it take for each gardener to do the weekly yard maintenance individually?

Answer 1

It takes the younger gardener about 49.11 minutes while it takes the older gardener about 77.11 minutes.

Explanation:

Let the time taken by the younger gardener = x minutes

`\text{The rate at which the young gardener will work}=(1)/(x)`

The older gardener takes 28 minutes more than the younger gardener, therefore:

The time taken by the older gardener = (x+28) minutes

`\text{The rate at wh}\imaginaryI\text{ch the older gardener w}\imaginaryI\text{ll work}=(1)/(x+28)`

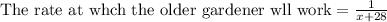
If they work together, it takes 30 minutes.

`\text{The rate working together}=(1)/(30)`

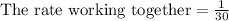
Therefore:

`(1)/(x)+(1)/(x+28)=(1)/(30)`

We solve the equation for x:

We then solve the quadratic equation for x using the quadratic formula:

`$$x=(-b\pm√(b^2-4ac) )/(2a)$$`

In our equation:a=1, b=-32, and c=-840

`\begin{gathered} $$ x=(-(-32)\pm√((-32)^2-4(1)(-840)))/(2*1) $$ \\ =(32\pm√((-32)^2-4(1)(-840)))/(2) \\ =(32\pm√(4384))/(2) \\ \implies x=(32+√(4384))/(2)\text{ or }x=(32-√(4384))/(2)\text{ } \\ x=49.11\text{ or x=-17.11} \end{gathered}`

Since time cannot be negative: x=49.11 minutes.

Thus, the time it takes:

• The younger gardener = 49.11 minutes

,

• The older gardener = 77.11 minutes

It takes the younger gardener about 49.11 minutes while it takes the older gardener about 77.11 minutes (correct to 2 decimal places).