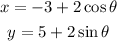Question

Which of the following sets of parametric equations represents (x + 3)2 + (y − 5)2 = 4?

Answer 1

`\begin{gathered} x=-3+2\cos \theta \\ x=5+2\sin \theta \end{gathered}`

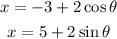
Explanations

Given the parametric equations represented by (x + 3)^2 + (y − 5)^2 = 4.

We need to determine the parametric equation that represents the equation. Checking the first parametric equation

`\begin{gathered} x=-3+2\cos \theta \\ y=5+2\sin \theta \end{gathered}`

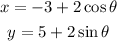
Substitute the parametric equation into the left-hand side of the parametric equation as shown:

`\begin{gathered} (-3+2\cos \theta+3)^2+(5+2\sin \theta-5)^2 \\ =(2cos\theta)^2+2(\sin \theta)^2 \\ =4\cos ^2\theta+4\sin ^2\theta^{} \\ =4(\cos ^2\theta+\sin ^2\theta) \end{gathered}`

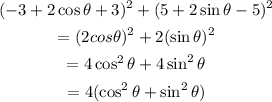
Recall from trigonometry identity that:

`\begin{gathered} \cos ^2\theta+\sin ^2\theta=1 \\ \text{Hence;} \\ 4(\cos ^2\theta+\sin ^2\theta)=4(1)=4(RHS)_{} \end{gathered}`

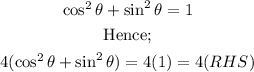
Since the result gave the right-hand side of the equation on simplification hence the correct sets of parameters equation are:

`\begin{gathered} x=-3+2\cos \theta \\ y=5+2\sin \theta \end{gathered}`