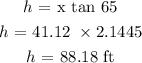Question

The accompanying diagram shows the plans for acell-phone tower that is to be built near a busyhighway. Find the height of the tower, to thenearest foot.Tower6531326100 ft

Answer 1

The height of the tower is 88 ft

Here, we want to get the height of the tower

We proceed as follows;

Let us represent this height by h

We can also have the full distance of the base as (100 + x)

What this mean is that for the triangle that contains the height and the angle 65, the base is x

Now, we can use the appropriate trigonometric identities to link up

With the angle 65, the tower is the opposite, while x is the adjacent

We use the tangent here;

`\begin{gathered} \tan \text{ 65 = }(h)/(x) \\ h\text{ = xtan65} \end{gathered}`

Furthermore, we have it that the base (100+x) is the adjacent and the height h is the oppsoite for the triangle that contains the angle 32

Thus, we have it that;

`\begin{gathered} \tan \text{ 32 = }\frac{h}{100\text{ + x}} \\ \\ h\text{ = tan 32(100+x)} \end{gathered}`

From here, we equate the two h values;

`\begin{gathered} x\text{ tan 65 = tan 32(100+x)} \\ 2.1445x\text{ = 0.62487(100+x)} \\ 2.1445x\text{ = 62.487+0.62487x} \\ 2.1445x-0.62487x\text{ = 62.487} \\ 1.51963x\text{ = 62.487} \\ x\text{ = }(62.487)/(1.51963) \\ \\ x\text{ = 41.12 ft} \end{gathered}`

To get the value of h, we simply substitute;

`\begin{gathered} h\text{ = x tan 65} \\ h\text{ = 41.12 }*2.1445 \\ h\text{ = 88.18 ft} \end{gathered}`