Question

The length of a rectangular piece of land is 60 yards more than two times its width. The perimeter is 540 yards. Find its dimensions.

Answer 1

According to the problem the length of the land is 60 yards more than two times the width, in a math expression this would be:

`\text{l = 2}\cdot w+60`

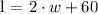
Where "l" is length and "w" is width. The perimeter of a rectangle is twice the length plus twice the width. Therefore:

`2\cdot l+2\cdot w\text{ = 540}`

Applying the first expression we can isolate one variable as shown below.

`\begin{gathered} 2\cdot(2\cdot w+60)+2\cdot w\text{ = 540} \\ 4\cdot w+120+2w=540 \\ 6\cdot w=540-120 \\ 6\cdot w=420 \\ w=(420)/(6) \\ w=70 \end{gathered}`

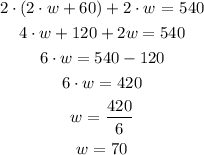
The width is 70 yards. We can use the first expression to find the length.

`\begin{gathered} l\text{ = 2}\cdot70+60 \\ l=140+60 \\ l=200 \end{gathered}`

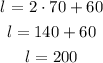
The length is 200 yards.