So we have the following piecewise function:

And we need to find f(-5). First of all we must decide which of the two expressions we have to use. We have x=-5 which is smaller than x=3 so we are in the interval x<3 which means that we must take the first expression and substitute x with -5:
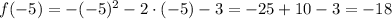
Then the answer is -18.