Question

Suppose a monochromatic light incident on a single slit produces a diffractionpattern.(a) How would the pattern differ if you decrease the wavelength of the light byhalf?(b) How would the pattern differ if you tripled both the wavelength and slit widthat the same time?

Answer 1

Given

Suppose a monochromatic light incident on a single slit produces a diffraction

pattern.

To find

(a) How would the pattern differ if you decrease the wavelength of the light by

half?

(b) How would the pattern differ if you tripled both the wavelength and slit width

at the same time?​

Step-by-step explanation

The fringe width is given by

`\beta=(\lambda D)/(d)`

where D is the distance between the slit and the screen, d is the width of the slit.

a. If the wavelength is decreased by half then the fringe width decreases by half. So teh distance between the consecutive bright spot of dark spot is reduced to half.

b. When the wavelength and the slit width would be tripled then the fringe width is

`\beta^(\prime)=(3\lambda D)/(3d)=(\lambda D)/(d)=\beta`

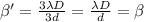
Thus the fringe width remains same. So the diffraction patternn remains same.