Given
system of equations:

Find
Solutions of the system of equations
Explanation
as we have given

so , from both equations , we obtain

now , solve the quadratic equation ,
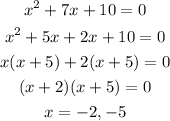
so , when x = -2 then ,

when x = -5 then
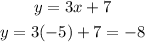
Final Answer
Hence , the solutions of the system of equations are (-2 , 1) and (-5 , -8). So , the correct option is B