Question

1.) A triangle with an area of 3 mm^2 is dilated by a factor of 6. What is the area of the dilated triangle? a) 9 mm²b) 12 mm²c) 18 mm²d) 108 mm²2.) A rectangle withA rectangle with an area of 5/8 ft² is dilated by a factor of 8.What is the area of the dilated rectangle? {DO NOT LEAVE YOUR ANSWER AS A FRACTION}3.) A prism with a volume of 270 m³ is dilated by a factor of 3.What is the volume of the dilated prism?4.) A prism with a base area of 8 cm² and a height of 6 cm is dilated by a factor of 54.What is the volume of the dilated prism?5.) A prism with a volume of 3125 ft³ is scaled down to a volume of 200 ft³.What is the scale factor?

Answer 1

Question 1:

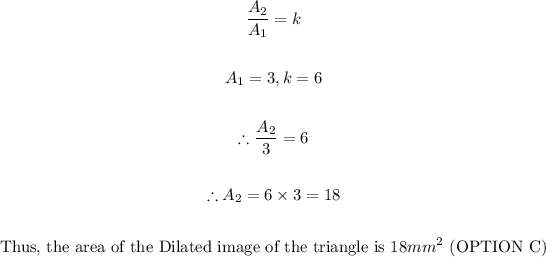
- The relationship between an area and its dilated image is given by:

`\begin{gathered} (A_2)/(A_1)=k \\ \text{where,} \\ k=\text{ Dilation factor} \\ A_1=\text{Area of the original object} \\ A_2=\text{Area of the Dilated image} \end{gathered}`

- We have been given the original area to be 3 and the dilation factor is 6.

- Thus, we can solve the question as follows:

`\begin{gathered} (A_2)/(A_1)=k \\ \\ A_1=3,k=6 \\ \\ \therefore(A_2)/(3)=6 \\ \\ \therefore A_2=6*3=18 \\ \\ \text{Thus, the area of the Dilated image of the triangle is 18}mm^2\text{ (OPTION C)} \end{gathered}`

Question 2:

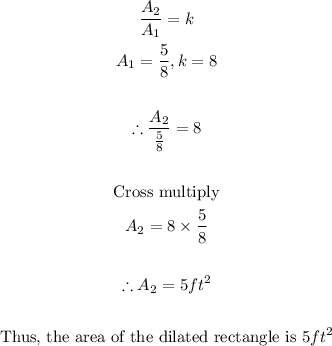
- The relationship described above also applies here, we have that:

`\begin{gathered} (A_2)/(A_1)=k \\ \text{where,} \\ k=\text{ Dilation factor} \\ A_1=\text{Area of the original object} \\ A_2=\text{Area of the Dilated image} \end{gathered}`

- The original area is 5/8 ft² and the Dilation factor is 8.

- Thus, we can solve the question as follows:

`\begin{gathered} (A_2)/(A_1)=k \\ A_1=(5)/(8),k=8 \\ \\ \therefore(A_2)/((5)/(8))=8 \\ \\ \text{Cross multiply} \\ A_2=8*(5)/(8) \\ \\ \therefore A_2=5ft^2 \\ \\ \text{Thus, the area of the dilated rectangle is 5}ft^2 \end{gathered}`

Final Answer

Question 1:

The area of the Triangle is 18mm²

Question 2:

The area of the Rectangle is 5ft²